I pride myself on giving students clear pathways to successful learning and maintaining an organized course structure. Please feel free to peruse the sample materials below that I have used in the past while teaching high school physics and college chemistry.
Sample Materials
Mario Kart 64 Lab
When I teach mechanics as part of an algebra-based physics course, I like to use this short activity to get students more interested in motion graphs (position/velocity/acceleration vs. time), a traditionally dry topic. The in-game speedometer of Mario Kart 64 (that was taken out of later games in the series) provides enough information for students to figure out the distance between the starting line and a wall. I like how this activity helps students synthesize an answer from several topics: kinematics equations, unit conversion, motion graphs, and multi-part 1D motion. And who doesn’t enjoy a casual (but still competitive) game of Mario Kart on the in-class projector?

Math/Circuits Practice Problems
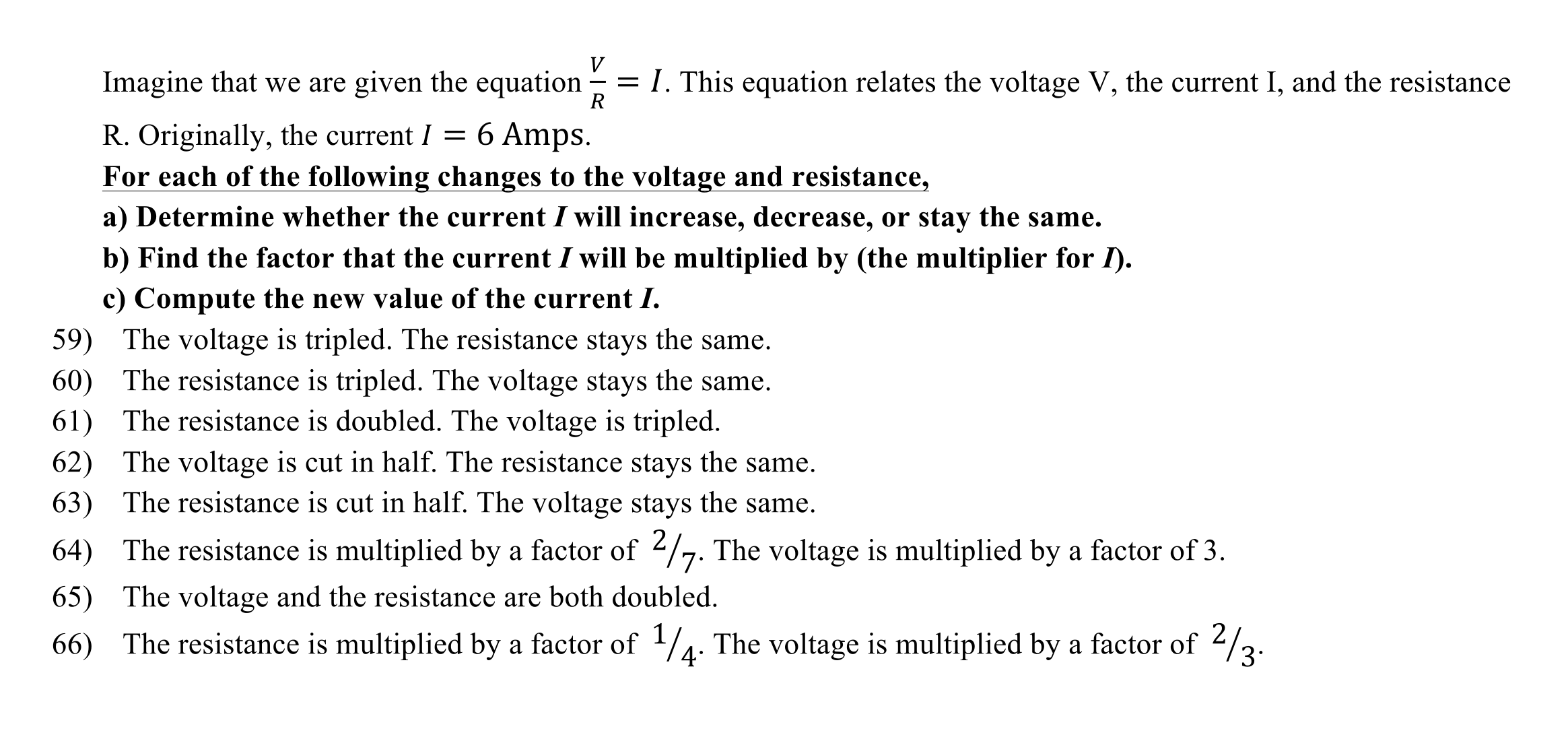
During the first year that I taught high school physics, many of my students struggled with test questions that relied too heavily on background prerequisite math knowledge, such as proportional reasoning and solving algebraic equations. For the next year, I generated a set of practice problems that focused on these common math stumbling blocks to help my students reactivate their knowledge from past math classes and transfer it to the new context of physics. Before diving into physics content in the next year, students worked through these problems in small groups and together with me at the board during a new math review unit tailored to the course. Questions #59-66 (from Sub-Unit 1-1: Mathematical Thinking) pictured here exemplify how this unit bridged students’ past knowledge of direct and inverse proportions with the context and question wording of my physics course.
Ensuring that students were confident in using proportional reasoning to analyze new equations, such as Ohm’s Law in this example, allowed me to spend more time during the rest of the course helping my students grapple with challenging physics concepts in lieu of math review, such as Question #22 (from Sub-Unit 6.1: Series and Parallel Circuits) shown here.
Organic Chemistry Review Session
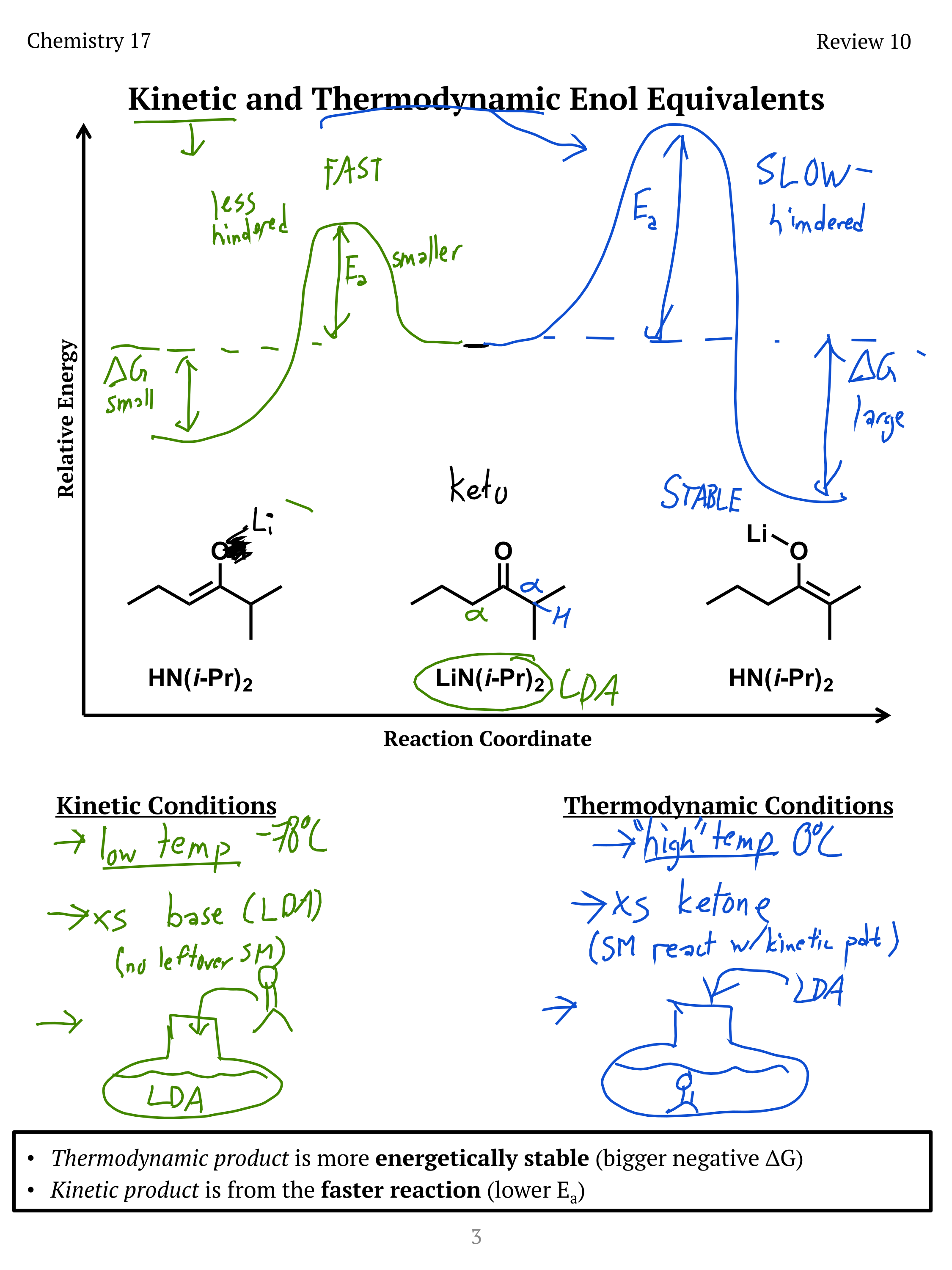
In addition to teaching two discussion sections of sixteen students each during Fall 2016, I was given the responsibility of preparing for and leading weekly, 90-minute, recorded review sessions for the entire course of 260 students. Each week I handed out blank review sheets for students to fill in with me as I worked through the notes and problems on the projector/tablet and took student questions throughout. I made sure to use color-coding to draw distinctions between concepts and to summarize key points in boxes at the bottom of the page when appropriate. The excerpt shown here is from near the beginning of Review Session 10, where I went over a key concept from that week (kinetic vs. thermodynamic products and their corresponding reaction conditions) using several different representations. After going over the key concepts in this “notes” style, the remaining time was spent working practice problems together.
One of the most challenging aspects of hosting these review sessions was choosing the correct pacing to go through the review packet. When students gave feedback at the midpoint of the semester, many expressed frustration about spending too long on content review and not enough time on problem solving, as I sometimes ran out of time before going over the last practice problem. To alleviate their concerns during the second half of the course, I shortened both the time and page space allotted to concept review and kept a closer look at the clock, allowing students to get more guided practice with the material without running over time.

Quantum Mechanics Exam Problem
While I was a graduate teaching assistant for an intensive general chemistry course in Winter 2018, I volunteered to write a question for one of the midterm exams. One of the topics covered in this course was the basic properties of quantum mechanical wavefunctions and how to use them to compute probabilities. I developed this question as a way to conceptually probe students’ understanding wavefunctions without requiring intensive computation.
Using Bloom’s taxonomy, the scaffolding of this question is as follows. Part (a) is an Understand-level question as the particle is most likely to be found where the square of the wavefunction has the greatest magnitude. Part (b) is an Apply-level question as integrating the square of the wavefunction, not the wavefunction itself, gives the numerical probabilities. Part (c) requires students to Synthesize several concepts, such as the symmetry of the problem, normalization of the wavefunction, and concepts from part (b). Even though this part technically involves integrals, students can use arithmetic to compute the answer and do not need to solve the integral by hand. Part (d) is mostly a Knowledge-level question of dimensional analysis.
By scaffolding the question in this way and not requiring intensive computations, we were able to decouple students’ conceptual understanding of wavefunctions from their mathematical ability. Two years later, I used a similar premise to propose a Teaching-as-Research project that would track how student participation in optional, online math review units would affect exam performance in an upper-level college physics course.
